메가슬롯 홈페이지

메가슬롯 공식 홈페이지로 연결되고 싶으시면, 페이지 상단의 배너나 바로가기 버튼을 활용하세요.
홈페이지의 상단 왼쪽에 배치된 이벤트안내 탭을 통해, 현재 진행 중인 이벤트를 간편하게 조회할 수 있습니다.
그 외에도 사용자는 잭팟 금액을 실시간으로 확인하고, 입출금을 처리하며, 쿠폰 발급을 받고, 자금을 이체하는 등의 다양한 기능을 사용할 수 있습니다.
홈페이지 최하단에는 텔레그램 및 위쳇 고객센터 정보가 있으니 이용에 문제가 생길 경우, 해당 채널로 문의하시면 됩니다.
무료 메가슬롯 머신 게임 목록
공식 홈페이지 하단부에서 메가슬롯과 정식으로 계약하고 있는 40개 이상의 다양한 슬롯 게임사들의 리스트를 제공합니다.
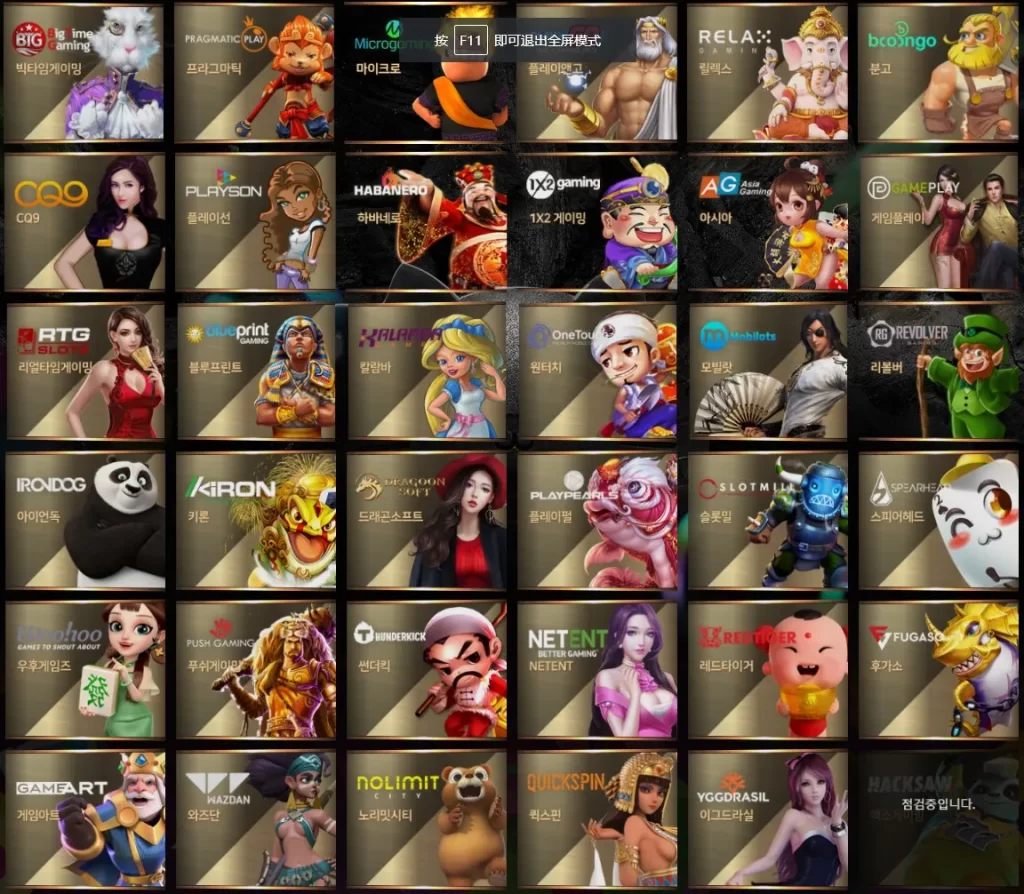
- 플레이앤고
- 하바네로
- 원터치
- 후가소
- 분고
- 핵소게이밍
- 원터치
- 키론
- 모빌랏
- 레드타이거
- 키론
- 모빌랏
- 블루프린트
- 칼람바
- 프라그마틱
- 분고
- 아리스토클랏
- 릴렉스
- 이그드라실
- 릴렉스
- 비비게임즈
- 게임플레이
- 우후게임즈
- 플레이앤고
- NETENT
- 아이언독
- 하바네로
- 아리스토클랏
- 하바네로
- 레드레이크
- 키론
- 키론
- 판타즈마
- 스프리트락
- 노리밋시티
- 프라그마틱
메가슬롯 이벤트

프리스핀데이 이벤트
메가 프리스핀 데이 이벤트가 매주 수요일에 열리게 됩니다
- 매주 수요일 활동내역별 프리스핀데이 쿠폰지급(해당 주 월요일, 화요일 활동내역)
- 고객센터 채팅창으로 이벤트 신청 후 이용할 게임 확인 / 본사에서 정한 슬롯게임만 이용 가능
- 프리스핀 쿠폰은 수요일 하루만 사용 가능
- 최대 출금 3배, 롤링 3배
- 프리스핀 쿠폰은 절대 입금액과 동시에 사용 금지, 사용 시 전액 몰수 처리
메가슬롯 신규가입 이벤트
메가슬롯 신규가입 시 아래와 같이 가입첫충 보너스가 지급됩니다.
신규가입 첫충전 보너스 20% + 1만 쿠폰(최대 100만)
가입 후 1회 입금 20%, 2회 10%, 3회 5% 보너스 지급
매일 첫입금 보너스 10%(최대 100만)
매일 재입금 보너스 5%(최대 100만)
레벨별 롤링콤프
등급 / 롤링콤프 / 조건 / 첫충보너스
- 레드 / 0.1% / 가입시 적용 / 신규첫충 20% 이후 10%
- 실버 / 0.1% / 누적충전 1천만원 이상 / 첫충 10%
- 골드 / 0.2% / 누적충전 1억원 이상 / 첫충 10%
- 플래티눔 / 0.25% / 누적충전 4억원 이상 / 첫충 10%
- 에메랄드 / 0.3% / 누적충전 8억원 이상 / 첫충 11%
- 루비 / 0.4% / 누적충전 15억원 이상 / 첫충 12%
- VIP / 0.5% / 누적충전 25억원 이상 / 첫충 15%
- VVIP / 0.6% / 누적충전 60억원 이상 / 첫충 15%
첫충 보너스 최대 100만
일요일마다 만 원 이상에서 천 원 단위로 콤프를 사용할 수 있습니다.
메가슬롯 지인추천 이벤트
추천인 수에 따라 아래와 같이 쿠폰 지급
- 추천인 3명 – 6만원 쿠폰
- 추천인 5명 – 10만원 쿠폰
- 추천인 10명 – 20만원 쿠폰
추천인으로 한 명을 인정받으려면, 최소 세 번 입금하고 총 입금액이 50만 원 이상이어야 합니다
추천인당 0.1% 배팅롤링콤프 영구 적용!
[주의사항]
- 매주 수요일 마다 1:1 문의로 추천인 쿠폰 요청시 발급
- 여러 계정의 사용 또는 같은 IP에서의 접속이 적발되면, 머니가 몰수됩니다
- 쿠폰만 사용 시 롤링 300%, 최대출금 3배까지 지급
- 이벤트 및 보너스 악용 회원은 게임 이용 제재
- 이벤트 기간 동안 이벤트 내용은 변경될 수 있습니다.
텔레그램 공식채널 이벤트
메가슬롯 공식채널에 참여한 모든 회원들에게 2만 쿠폰 지급!
정보와 공지를 빠르게 받아보기 위해 공식 채널 가입을 추천합니다.
[이벤트 참여 방법]
- 고객센터를 통해 공식채널 링크 문의
- 공식채널 입장 후 고객센터로 스크린샷 첨부
- 채널 이벤트 2만 쿠폰 확인

메가 슬롯 : 모두의슬롯

여러분은 온라인 슬롯 게임의 세계로 스릴 넘치는 여행을 시작할 준비가 되었나요? 여러분의 인생을 바꿀 수도 있는 거대한 상품들의 약속으로 릴이 회전하고 있는 메가슬롯만 보아도 됩니다! 고품질의 그래픽, 혁신적인 기능, 그리고 풍부한 흥분이 결합된 몰입형 게임 경험에 사로잡힐 준비를 하세요. 그것은 큰 승리와 수익성 있는 보상을 찾기 위해 릴을 굴릴 때 여러분의 내적 모험가를 분출할 수 있는 기회를 제공합니다.
다양한 주제와 흥미로운 보너스 라운드로 모든 스핀은 기대와 잠재적인 부를 담고 있습니다. 클래식한 과일 슬롯을 좋아하거나 현대적인 비디오 슬롯에 매료되었다면 모든 취향을 만족시킬 게 있습니다. 이미 전 세계의 많은 플레이어들이 그 흥미를 발견했으니, 당신도 대담한 아드레날린 세계에 참여하세요.
메가슬롯을 플레이하는 것은 현재의 큰 보상을 얻을 수 있는 흥미로운 기회를 제공하는 즐거운 경험입니다. 먼저 원하는 장치에서 손쉽게 게임을 시작하세요. 목표는 릴 전체에서 일치하는 기호를 사용하여 승리 조합을 만드는 것입니다. 이를 위해 게임의 작동 방식을 이해해야 할 것입니다. 일반적으로 다섯 개의 릴과 여러 개의 페이라인 또는 승리 방법으로 구성됩니다.
메가슬롯 먹튀 | 슬롯 가입쿠폰, 지금 당신의 것으로 얻으세요!

릴을 돌리기 전에 베팅 크기를 기호에 따라 조절합니다. 그런 다음 스핀 버튼을 누르고 기호가 무작위로 나타나는 것을 주시합니다. 와일드나 산점과 같은 특별한 기호를 주의하고, 자유 스핀이나 미니 게임과 같은 흥미로운 보너스 기능을 활성화할 수 있습니다. 몇몇 메가슬롯은 무작위로 또는 특정 기호 조합으로 얻을 수 있는 점진적인 잭팟을 제공합니다.
메가슬롯의 세계에서 플레이어들은 릴이 돌아가는 흥미와 함께 엄청난 보상을 얻을 수 있는 기회로 인생을 현실로 바꿀 수 있습니다. 캐시 잭팟부터 이국적인 여행지에서의 풍부한 휴가까지 플레이어들을 위한 다양한 수익성 있는 보상을 제공합니다.
신입자든 베테랑 플레이어든 항상 놀라운 것이 있습니다. 하지만 그것은 단지 큰 승리에 관한 것이 아닙니다 – 그것은 모든 플레이어가 보상을 받을 수 있는 기회를 보장합니다. 다양한 보너스 기능과 게임 플레이에 포함된 미니 게임으로, 더 작은 승리도 합쳐지고 아주 신나는 경험을 만들 수 있습니다. 그러므로, 다른 어떤 것들과 같은 모험을 준비하세요.
투데이슬롯

게임은 매끄러운 조작감을 통해 플레이어가 게임 내에서 완벽하게 몰입할 수 있게 해줍니다. 돋보이는 비주얼과 매혹적인 애니메이션으로 모든 순간이 생생하게 다가오며, 게임 플레이의 질을 향상시킵니다. 보너스 라운드와 프리 스핀 같은 기능들로 인해 더 많은 승리 기회를 제공합니다.
메가슬롯은 보너스 라운드와 특별 기능을 통해 플레이어에게 승리 가능성을 높여주는 동시에 짜릿한 게임 경험을 제공합니다. 특히, 와일드 기호는 게임에서 중요한 역할을 하며, 다른 기호를 대체할 수 있는 독특한 로고로, 승리 조합을 얻을 확률을 증가시킵니다. 스캐터 기호 역시 게임의 재미를 배가시키는 보너스 라운드를 유발할 수 있어 주목할 가치가 있습니다.
메가슬롯에서 제공하는 프리 스핀 기능은 게임을 더욱 흥미롭게 만드는 추가 요소입니다. 스캐터 기호를 세 개 이상 맞추면 무료 스핀이 활성화되어 모든 승리가 배로 증가합니다. 이는 플레이어에게 추가 비용 없이 큰 보상을 얻을 수 있는 절호의 기회를 제공합니다. 게다가, 보너스 라운드에는 독특한 미니 게임이 포함되어 있어, 승리를 위한 전략적 사고가 요구됩니다.
메가 카지노 FAQ 자주 묻는 질문
메가슬롯에서 다른 카지노나 스포츠 베팅도 지원하나요?
아니요, 메가슬롯은 슬롯 게임만을 전문으로 취급하는 사이트로, 정품 게임만을 다루고 있습니다. 슬롯 게임의 다양성과 품질 모두에서 뛰어난 퀄리티를 자랑합니다.
메가슬롯 궁금한 점이나 문제가 생겼을 때 어디에 문의하면 되나요?
공식 홈페이지 바닥글에 있는 고객센터 계정을 통해 질문하실 경우, 하루 24시간 언제든지 빠르게 답변 드릴 것입니다. 그리고 텔레그램 공식 채널 가입 이벤트도 있으니 혜택을 확인해 보시길 권장합니다.

